Feynman Sprinkler

December 31st, 2011 21 Comments

Perhaps you have heard of the Feynman Sprinkler before; it’s a relatively famous physics conceit, though admittedly, physics mind-benders are not necessarily part of the public consciousness. Feynman himself was particularly coy when writing about it in his autobiography, abstaining from sharing the result of his experiment, which has led to the typical internet speculation (see: airplane on a treadmill). It’s such a neat problem because smart, informed people can come to completely different conclusions as to what happens when you run a sprinkler in reverse.


Normal Sprinkler		Feynman Sprinkler

Obviously, our “normal” sprinkler will spin clockwise. Feynman wanted to know what direction it would spin when run in reverse. I think there’s a general consensus that an underwater sprinkler, when run in reverse will not spin, but why? I’ve heard various explanations that are either confusing, misleading, or wrong, so I wanted to tackle it myself.

What’s the big problem anyway? Why is this so hard to wrap your head around? I think the core of the confusion is that we have a problem which appears to be symmetric, and in physics, we expect symmetric situations to behave symmetrically. But, in fact, the symmetry is an illusion. Why? We’ll get to that…

First, I’d like to begin the analysis with our trusty friend, the control volume. Again, we have to be careful; this analysis has given me considerable trouble due to silly assumptions that I’m used to making, but cannot be made here.

All right, first let’s start with the easier case: that of a sprinkler running in the “correct” direction underwater. To simplify the problem, we will assume the sprinkler is not moving or accelerating or anything. Speaking of symmetry, we’re going to use it here, and only examine half of our sprinkler:

Here’s a cross-section of the sprinkler; so let’s set up a control volume around the outside of the sprinkler pipe, across the entrance and exit. Here’s our scary-looking equation:

$\sum\left( \vec{r} \times\vec{F}\right)_{tot}=\frac{\partial}{\partial t}\int_{cv}\left( \vec{r} \times\vec{V}\right) \rho dV+\int_{cs}\left( \vec{r} \times\vec{V}\right) \rho\vec{V}\cdot \hat{n}dA$

Okay, let’s start rocking the terms. Since we only care about the static state, we can drop the time-dependent term:

$\sum\left( \vec{r} \times\vec{F}\right)_{tot}=\int_{cs}\left( \vec{r} \times\vec{V}\right) \rho\vec{V}\cdot \hat{n}dA$

Because I’ve set up the problem so elegantly, everything happens at right angles and the cross products can simplify so that we are simply balancing torques perpendicular to the page:

$\tau_{tot}=\rho A_{in}RV^{2}=\rho QRV=\dot{m}RV$

The first term is equivalent to the total torque being applied to the control volume, while the second term comes from evaluating the integral for the flow out of the nozzle (top of the diagram). This is the only flow in our problem happening at any distance from the pivot point. The inflow is occurring at the axis of rotation and so it doesn’t contribute anything to the fluid’s angular momentum about that point.

Our job is now to equate the total torque with the torques on the control volume’s surface. Anything left over must be due to the external torque needed to keep the sprinkler still while water is flowing through it.

$\vec{\tau}_{tot}=\vec{\tau}_{surf}+\vec{\tau}_{ext}$

Generally, the torque is calculated by integrating $\vec{r}\times\vec{F}$ over the control surface, where $\vec{F}$ is determined by $p\hat{n}dA$ . It so happens for this type of finite control volume analysis, it works best to assume that the nominal fluid pressure is zero. This “gauge” pressure can be set arbitrarily without affecting our results, so for convenience (and to reduce the number of integrals we have to do), we will set it to zero, and fluid pressures will all be relative to this. That is, all of the surrounding, unmoving fluid in the problem will have a pressure of zero. Furthermore, in fluid mechanics, a “free jet” such as the one we have here, has a pressure equivalent to the surrounding fluid (that is, zero).

Soooo…. that means the pressure, and therefore the force all around the control volume is zero; which means $\tau_{tot}=\tau_{ext}$ and we have

$\tau_{ext}=\dot{m}RV$

So the torque required to keep the nozzle in place is $\dot{m}RV$ (positive, out of the page). Another way to look at it is that this half of the nozzle apparatus (everything in the control volume) is exerting a torque of $-\dot{m}RV$ on the hub, or whatever is holding it in place. Doing the same analysis on the other half would yield the same result, with the additive, total torque required to keep the sprinkler still equal to $2\dot{m}RV$ .

Have I lost you yet?

Okay, then; let’s keep going.

Here we have the case of the reverse (Feynman) sprinkler. It seems like a straight-forward process to redo the calculations, but with opposite flow conditions, but unfortunately some of our more essential assumptions break down. I think this is what creates confusion — a lack of symmetry in the flow.

A low-hanging fruit is that the pressure of the inflow is not equivalent to ambient in the reverse case. This can be worked out using the Bernoulli equation to determine pressure as a function of velocity, where along a streamline, (specific) energy is conserved:

$\tfrac{1}{2}\rho V^2_1+\rho g z_1 + p_1=\tfrac{1}{2}\rho V^2_2+\rho g z_2 + p_2$

Since the fluid is being accelerated from the quiescent surroundings (which are at zero pressure), and change in elevation is negligible, the equation becomes:

$0=\tfrac{1}{2}\rho V^2_2 + p_2$
$p_2 =-\tfrac{1}{2}\rho V^2_2=\tfrac{1}{2A_{noz}}\rho Q V_2$

All right, I haven’t been entirely upfront with you. This is not a great application of the Bernoulli equation.

Where did we/I go wrong?

My cartoons have not been doing the flow field justice. The velocities at the exit probably look closer to this (regular sprinkler on the left, reverse sprinkler on the right):

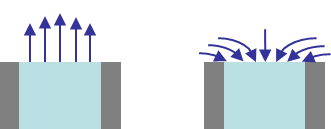Another illustration of the non-intuitive asymmetry of the problem

The outflow velocities can more or less be considered to be parallel, and have an either laminar or turbulent profile, resulting in our expression for torque being mostly unchanged. However, I don’t have any good analytic expression for the velocity profile coming in, making our control volume analysis exceedingly difficult (I know there’s a vena contracta and separated flow as the fluid enters the nozzle). Since we’re assuming the mass flow rates are the same, though, I’ll take a leap of faith and say that right hand side of the control volume equation is the same in both cases (the velocity is the opposite direction, but the sign stays the same because it is squared).

But let’s use our new information to do a better job with the Bernoulli equation. When streamlines are bent so severely, some of the Bernoulli assumptions about conserved energy (inviscid-ness) are violated. This energy loss can be accounted for by introducing a head loss term:

$\tfrac{1}{2}\rho V^2_1+\rho g z_1 + p_1=\tfrac{1}{2}\rho V^2_2+\rho g z_2 + p_2+h_L$

where

$h_L=\tfrac{1}{2}K_L \rho V^2$

What of this $K_L$ ? It is a loss coefficient that is a function of the geometry of the loss feature; in our case the nozzle entrance. Similar “re-entrant” pipes tend to have very high coefficients, approaching the maximum of unity. Supposing it is unity, let’s recalculate the pressure $p_2$ :

$0=\tfrac{1}{2}\rho V^2_2 + p_2+h_L=\tfrac{1}{2}\rho V^2_2 + p_2+\tfrac{1}{2}\rho V^2_2$
$p_2=\rho V^2_2=\frac{\rho Q V_2}{A_{noz}}$

I’m sick of caveats, but here’s another one. The pressure is not uniform across the entrance, nor do the losses happen at the entrance. The pressure drop actually occurs due to viscous effects in the separated flow along the entrance of the pipe; but since we’re assuming inviscid flow, this is the correction we need to apply to the upstream pressure (at the entrance) to overcome these viscous losses.

We can then determine the torque from surface forces:

$T_{surf}=R p A_{noz}$
$T_{surf}=R \rho Q V$
$T_{surf}=\dot{m}RV$

And now to work it in to our full control volume equation:

$T_{tot}=T_{ext}+T_{surf}=\int_{cs}\left( \vec{r} \times\vec{V}\right) \rho\vec{V}\cdot \hat{n}dA$
$T_{ext}+\dot{m}RV=\dot{m}RV$
$T_{ext}=0$

So that means there are no outside torques needed to keep the nozzle in place; in other words, the nozzle is not going to spin even if we don’t hold it.

Do I have you convinced?!

Although Feynman never revealed “the answer” or what happened when he tested it out in the lab, the general consensus is that there is no torque on the reverse sprinkler. However, knowing the answer definitely helped me get here, and I’m not sure that I can be totally confident in my assumptions. That being said, the flow profile, and therefore the analytical solution, is pretty messy in the reverse case, and requires some bold assumptions to make the problem tractable. Even if this explanation is not a concrete proof, I hope it helps align the math of the problem with experimental results. It certainly helped me rationalize the phenomenon.

Posted in Feynman |

You can leave a response, or trackback from your own site.

21 Responses to “Feynman Sprinkler”

Shaz says:

January 16, 2012 at 9:49 pm

I’ve waited a long time for this one. I’m thoroughly impressed.

Reply
K-Mob says:

January 28, 2012 at 9:03 pm

Does that mean it makes sense?!

Reply
- Shaz says:
  
  January 30, 2012 at 7:50 pm
  
  I didn’t work it out myself, but it all seems highly plausible. I find your addressing the velocity profile at the inlet appropriate. It is a subtle point that is likely more significant than it would seem at first glance.
  
  Good work!
  
  Reply
Chuck Johnson says:

March 15, 2012 at 9:48 pm

A Feynman sprinkler using air instead of water is shown on Youtube. Follow this link:

http://tinyurl.com/83w6cab

The sprinkler turns and accelerates in the opposite direction of a sprinkler which ejects mass instead of pulling it in.

I know how this works and why it works. No math is needed to explain how it works.

Reply
- K-Mob says:
  
  March 20, 2012 at 4:47 pm
  
  Physics/math is very much needed if you hope to understand how it works (or doesn’t).
  
  Here is an MIT website that claims the opposite outcome:
  http://web.mit.edu/Edgerton/www/FeynmanSprinkler.html
  
  I guess the point isn’t exactly what the answer is, but that it is not in any way intuitive, and certainly the physics are not “the opposite” of the normal sprinkler case.
  
  Reply
  - Chuck Johnson says:
    
    June 11, 2012 at 2:10 pm
    
    “Although Feynman never revealed “the answer” or what happened when he tested it out in the lab, the general consensus is that there is no torque on the reverse sprinkler.”
    
    The statement above in quotes is not true.
    
    In his book “Surely You’re Joking, Mister Feynman” Richard Feynman reveals that the reverse sprinkler does produce torque. He observed this torque and reported it in his book. The dirction of the torque is not reported by Feynman, but two Youtube videos show that the torque is opposite to torque produces when fluid flows out of the nozzle.
    
    Here are those two Youtube videos: http://tinyurl.com/83w6cab
    Also: http://tinyurl.com/742r2kg
    
    The first is attributed to Henry Ariail. The second video was recently made by myself. I built the rotor shown in the video. The experiments by Feynman, Ariail, and Johnson all agree that the reverse sprinkler will produce torque and it will spin.
    
    None of these demonstrations give an exact figure for the magnitude of the torque produced. Feynman’s experimental apparatus exploded before he could get a good measurement. I can report that the torque produced by a reverse sprinkler is very weak. It required three vacuum cleaners all working together to make the rotor spin. It is obvious to me that the Feynman sprinkler demonstration displayed at MIT was not built with enough precision and sensitivity to show the very small force which is present.
    
    Why should the inflow rotor produce such a small amount of torque?
    That’s an interesting question, and the answer is also interesting.
    
    Reply
  - Chuck Johnson says:
    
    June 11, 2012 at 2:24 pm
    
    Here is the relevant passage from Richard Feynman’s book.
    Feynman uses a flexible rubber hose to indicate the presence of torque. He reports that the hose twisted in response to the flow of water. The twisting of the hose was used by Feynman to measure the magnitude of torque produced by the inflow rotor.
    
    By blowing air into the bottle, I could force water into the copper
    tubing exactly as if I were sucking it in. Now, the S-shaped tubing wouldn’t turn around, but it would twist (because of the flexible rubber hose), and I was going to measure the speed of the water flow by measuring how far it squirted out of the top of the bottle. I got it all set up, turned on the air supply, and it went “Puup!” The air pressure blew the cork out of the bottle. I wired it in very well, so it wouldn’t jump out. Now the experiment was going pretty good. The water was coming out, and the hose was twisting, so I put a little more pressure on it, because with a higher speed, the measurements would be more accurate. I measured the angle very carefully, and measured the distance, and increased the pressure again, and suddenly the whole thing just blew glass and water in all directions throughout the laboratory.
    
    Reply
    - K-Mob says:
      
      June 25, 2012 at 6:25 pm
      
      Thank you for sharing your video and the excerpt from the book. I actually found a pretty neat write-up off the Wikipedia page that gives another explanation from a different point of view; although he says that the torque is only present during flow transitions; once the flow and rotation are established, angular momentum is constant and no torque is exerted, which sits well with me (though upon cursory examination, your demonstration may refute that):
      
      http://arxiv.org/abs/physics/0312087
      
      Ultimately, in order for me to make the problem tractable, my “solution” has to make a lot of assumptions that cannot stand up to scrutiny when dealing with such subtle effects. To me, that’s what makes the problem so interesting. It’s deceptively difficult/complicated.
      
      Your coyness on your belief of what the “answer” is is a bit odd, though…
      
      Reply
      - Chuck Johnson says:
        
        June 25, 2012 at 7:24 pm
        
        K-Mob,
        My coyness stems from the fact that I enjoyed figuring this thing out, and I would like others (You, perhaps) to get it, too.
        
        So here are my next clues: (Remembering that the torque is much less than the torque observed in an outflow sprinkler), my solution does not deny the conservation of angular momentum. My solution requires that angular momentum must be conserved.
        
        My solution does not deny that “For every action there is an opposite and equal reaction” My solution is just one more affirmation of Newton’s third law of motion.
        
        Curiouser and curiuoser? – – – Maybe for the moment. – – – But give it some thought. The solution is really down to earth. I will give more clues as needed. Also, I would like you to imagine that it is possible that I really do have the correct solution. Think different.
      - Chuck Johnson says:
        
        July 7, 2012 at 5:59 pm
        
        K-Mob,
        I followed your link several days ago and I found very little useful information in the few sentences published there. The link takes you to the Cornell University Library. Today, I followed the link again, but this time I clicked on the Alejandro Jenkins name and this took me to a list of published papers. Jenkins has published an 11 page PDF here:
        
        http://arxiv.org/pdf/0908.3190.pdf
        
        You should read this PDF. It includes this line:
        
        There will be some amount of water flow that does not enter the sprinkler head.1 The corresponding angular momentum will be transferred to the surrounding tank, and, with respect to the tank’s frame of reference, the reverse sprinkler will experience a small torque even in the steady state. This torque tends to make the reverse sprinkler turn towards the incoming water, in the direction opposite to the rotation of the regular sprinkler.
        
        This comment by Jenkins agrees with my experimental results. This same comment gives the correct reason that an inflow sprinkler should generate continuous torque, and not just starting and stopping torque.
      - K-Mob says:
        
        July 9, 2012 at 4:18 pm
        
        Indeed, the link that I pointed to first was the page hosting this PDF:
        
        http://arxiv.org/pdf/physics/0312087v3.pdf
        
        Which is several iterations and 8 years previous to the one you pointed to. I agree with your conclusions, and specifically, the line you cited in the PDF. Perhaps my favorite line from the same paper, right after the line you quoted:
        In short, both the regular and the inverse sprinkler can turn only to the extent that angular momentum may be
        transferred to the surrounding environment
        
        You are absolutely right, that my analysis is only including the collected mass, which is why it fails to calculate a continuous torque (to be fair, even with the assumptions that I’ve made, the conclusion of no net torque is fairly shaky).
        
        You deserve credit for actually doing the experiment, as Feynman did.
        
        I’ll reiterate that the usefulness of my exercise was not to come down on either side of the aisle per se (though that was the motivation), but to demonstrate how difficult a problem it is compared to the “traditional sprinkler” we all learn about in fluid mechanics. I’ve never seen a complete analytical treatment, and I’ll go so far as to say I don’t think one exists.
        
        Thanks again for your input!
      - Chuck Johnson says:
        
        July 7, 2012 at 6:53 pm
        
        K-Mob,
        So here is a simple, clear explanation of the inflow sprinkler (Feynman sprinkler) effect:
        
        This rotor produces torque and spins continuously because some amount of mass (let’s call it uncollected mass) is attracted to the nozzle by suction, but that mass never enters the nozzle. This mass can be solid, liquid or gas.
        
        We can imagine that most of the fluid that responds to the suction and begins to move towards the nozzle ends up being collected by the nozzle. Collected mass does not contribute to Feynman torque. Only uncollected mass does. The majority of attracted mass gets collected. Only a small amount of mass gets attracted, but misses getting collected. The implication of this is that only a small amount of Feynman torque will be available and some experimenters (such as at the Edgerton Center) will overlook this small effect and they will come to the wrong conclusion.
        
        It seems that all of the math at the top of this page calculates for collected mass. Uncollected mass was not imagined.
        
        So this is how I and Alejandro Jenkins reconcile experiment and theory. Uncollected mass is the culprit. The Feynman sprinkler really does spin as long as suction is maintained. Again watch the Youtube video that I made. During the entire video, the airflow remains the same. All three vacuum cleaners are running continuously, and there are no transient effects present. The rotor accelerates under steady state mass flow conditions.
    - Chuck Johnson says:
      
      November 3, 2012 at 7:56 pm
      
      K-Mob,
      Now it occurs to me that Richard Feynman was not very smart to set up the experiment with a substantial amount of air in the bottle. Feynman reports that “I got it all set up, turned on the air supply, and it went “Puup!” The air pressure blew the cork out of the bottle.”
      
      That “Puup!” noise happened when compressed air ejected the cork, and this should have alerted Feynman to the amount energy stored in the bottle in the form of compressed air. If the bottle had little or no air in it, the experiment would have been much safer. The bottle should have been filled to the top with water. Pressurized water should have been pumped in, instead of compressed air. Water has very little compressibility, and it would not have stored enough energy to explode as Feynman has reported.
      
      Feynman must have known about the compressibility of air, and he must have known about the ability of compressed gases to store energy. The excitement of running the experiment probably made him forget these things.
      
      Reply
  - Chuck Johnson says:
    
    August 4, 2012 at 8:24 pm
    
    K-Mob,
    I figured out the solution without having to do any math calculations. I did use the concept of “equal”, however.
    
    I began by referring to Newton’s Third Law Of Motion. I used this approach because I knew that when an inflow sprinkler demonstrates Feynman torque, there must be a corresponding swirl or rotation of mass going in a direction opposite to the rotation of the sprinkler. If I could find the location of this counter rotating mass, I could solve the problem.
    
    I then did a thought experiment. I imagined a particle of mass (an air molecule) hovering near the inflow nozzle. I saw the particle accelerate towards the nozzle under the influence of suction, and I knew that the momentum gained by the mass particle would be matched by the exact same amount of momentum being imparted to the sprinkler. Each separate momentum would be in an opposite direction. Now we have a source of Feynman torque.
    
    I followed the particle into the nozzle and I watched it crash into the ninety degree bend in the sprinkler arm. This crash neutralized the momentum of the particle and the momentum of the sprinkler. The source of Feynman torque has now vanished. The result would be transient startup torque, or no torque at all. Either way, I had no source of continuous Feynman torque. Then I watched as the particle headed down the sprinkler arm towards the rotary coupling,and finally geting sucked out of the system.
    
    When mass (air molecules) exits the system through the rotary coupling, some angular momentum can exit along with the mass. This happens when the system is already rotating. It happens because the radius of the rotary coupling is greater than zero. With further thought, however, I realized that the angular momentum which exits is in the same direction as the rotation of the sprinkler. This will only slow the system down, and can never be a source of Feynman torque.
    
    At this point, I had chased the mass particle down a rathole. I knew that these particles of collected mass were useless to explain the generation of Feynman torque.
    
    I knew that somewhere in the universe, a rotation of mass must exist which spins opposite the direction of the Feynman sprinkler, and exactly accounts for the amount of momentum that the the sprinkler is receiving. The exploding experiment of Richard Feynman, the Henry Ariail demonstration, and the Chuck Johnson demonstration all showed that continuous Feynman torque can be achieved. I had chosen a gold standard of mechanical analysis, Newton’s Third Law. I just needed to find that swirl of mass particles.
    
    Going back to the thought experiment, I next saw a mass particle accelerate towards the nozzle, but just miss the edge of the nozzle. This would solve the problem if a significant amount of mass would miss getting collected. This idea was supported by an odd thing that I had noticed: when the sprinkler starts from a dead stop, it accelerates at a sluggish pace. As it picks up speed, it seems to accelerate more quickly. This would be exactly what I would expect. The tip of the nozzle starts moving past larger and larger amounts of mass which never gets collected and this causes Feynman torque to increase. Many air molecules get attracted by the suction and undergo acceleration, but many will miss getting collected because the nozzle tip has moved on before the air molecule gets all the way to the tip. The sprinkler eventually reaches a steady state maximum speed of rotation as bearing friction and air friction increase with increasing RPM.
    
    K-Mob, do you remember what Rod Serling used to tell us each week?
    
    “You unlock this door with the key of imagination.”
    
    Reply
    - K-Mob says:
      
      September 20, 2012 at 10:59 pm
      
      I like it!
      
      In fact, I believe that most of the arguments I’ve read argue for zero torque have assumed inviscid flow. Which of course is not the case (although that would be an interesting experiment if it were possible), and viscosity is required in the situation you envision to sling particles past the nozzle entrance.
      
      I do believe that’s a pretty tidy physical explanation.
      
      Reply
    - G Bouwman says:
      
      June 4, 2013 at 8:23 am
      
      Hi Chuck and K-Mob,
      
      I’ve been doing some experiments on this rather simple looking sprinkler. Though since there is a lot of literature about it, it is known to be a complex question. Now my results might be even more confusing since i got my sprinkler (same shape) moving in either both directions 🙂
      
      Greetings!
      
      Reply
Allan Rosenberg says:

June 4, 2012 at 2:57 pm

Thank you for proving mathematically that angular momentum is not conserved. I’m sure that Emmy Noether is rolling in her grave right now. Come to think of it, maybe that’s where the angular momentum of the fluid moving into the ends of the tube went.

Reply
- K-Mob says:
  
  June 25, 2012 at 6:29 pm
  
  My intention throughout the analysis was to ensure conservation of angular momentum. If you could point out a specific error, I’d be happy to address/correct it. On the other hand, if you are simply in the business of trolling physics posts on the interwebs, a tip of my cap to you for the Noether reference!
  
  Reply
  - Chuck Johnson says:
    
    July 14, 2012 at 7:56 pm
    
    It seems that Allan Rosenberg was bored with all of that math and decided to insert a joke.
    
    To see a practical consequence of the fluid dynamics discussed above, visit Youtube and view one of the videos showing the way that jellyfish move through the water.
    
    I used to be puzzled as to why a jellyfish is able to make continuous headway through the water by repeatedly taking in and then expelling sea water. The fluid dynamics discussed above help to show why the jellyfish can make continuous headway through the water.
    
    Reply
    - K-Mob says:
      
      July 21, 2012 at 1:41 pm
      
      Yes, that’s a great point. I’ve seen a couple different presentations on low-Reynolds number propulsion.
      
      Check out the “scallop theorem” proposed by Nobel prize winner Edward Purcell. It basically states that the motion cannot look the same forward and backwards in time in order for an organism in low-Reynolds number flow to make forward progress.
      
      This isn’t necessarily low-Re flow, but the idea is largely the same.
      
      Reply
Feynman at Caltech | 3 Quarks Daily says:

May 28, 2018 at 2:11 am

[…] been a lot like Faraday, in the sense that Faraday wanted to test everything in the lab first (as Feynman tried to do with the water sprinkler problem). Of all the famous Feynman quotes, it is this one below that most sums up for me his particular […]

Reply